Simple Interest Explained Clearly: Is it Simple?
You are probably fairly familiar with the words simple interest? The words simple interest pop up continuously in everyday life when you talk to your bank or walk by an advertisement that advertises a car loan or a credit card or other loans and financial services.
If you have savvy friends, they might even ask you to pay them simple interest when you borrow money from them.
What is simple interest? How is simple interest calculated? What is the simple interest formula? And last but not least how do you use simple interest and when?
All these questions and more will be answered in this article together with useful resources for you to practice on.
What is simple interest?
Simple interest is a charge or fee for borrowing money. Instead of having a monetary fee (like X amount of EUR / USD / GBP) you can instead say that the simple interest is X%.
This means that the cost to the borrower is the X% and the return / profit for the lender is the same X%.
Obviously the lower the simple interest the better off the borrower is and the higher the interest rate the better off the lender is.
Simple interest rate is thus the value of money at a given time and it is negotiated between the borrower and the lender. This interest changes over time and in fact it changes every day on the global markets but normal people would not see this change. In recent times like 2015-2017 the interest rates have been very low globally and have even been negative in some countries. This is not normal though and we will not discuss this topic here.
The point we are just making is that interest rates vary over time and from country to country so it is not a static number but instead it varies all the time.
What is the simple interest formula?
So, let’s immediately jump in the deep end and get more intimate with the simple interest formula. Trust me, it is a lot easier than you might think.

Simple Interest Formula:
A = P(1 + rt)
Where:
A = Total Accrued Amount (principal + interest)
P = Principal Amount (the amount borrowed)
r = Rate of Interest per year in decimal form; r = R/100
R = Rate of Interest per year as a percentage; R = r * 100
t = Time Period in months or years
You have various online resources and calculators as well to calculate simple interest and one good such source is here.
You can also check out this video lesson on simple interest which will explain it to you in detail:
Let’s use a quick example so you get a feeling for the formula before we look at it in more detail with more examples.
Example:
You borrow 1 000 from a personal finance lender and they tell you the interest rate is 4%/year. What is the simple interest amount and what is the total amount accrued at the end of year 1?
Simply put these values into the formula and you will have your answer in no time.
A = P(1 + rt)
A = 1 000(1 + 0.04*1)
Where 0.04 is 4%/100 and 1 is one year
A = 1 000(1.04)
A = 1 004
So, the answer is total amount of the loan plus interest i.e. total amount accrued is 1 004. The interest amount is thus 1 004 – 1 000 = 4.
You paid 4 in interest.
How is simple interest calculated?
Simple interest is actually very simple to calculate as you could already see from the previous example but in this section, we will use a few more examples to show you how to use the simple interest formula and how to calculate simple interest.
So first of all, let’s add the formula here to remind you how it looked like:
A = P(1 + rt)
Example:
You want to borrow money for a car you want to buy your parents. It is a bit expensive so you take a loan for 5 years with simple interest of 5%/year. The price of the car is USD 5 000. How much is the total amount to be paid back after year 5 and what is the total interest you are going to have to pay back?
By filling in the values in the simple interest formula you get this:
A = 5 000(1 + 0.05*5)
A = 5 000(1 + 0.25)
A = 5 000(1.25)
A = 6 250
So, you would pay back in total USD 6 250 in simple interest AND the borrowed amount.
The interest amount is thus 6 250 – 5 000 = USD 1 250.
In other words because you borrowed money to buy the car the cost of the car just went up by 25%!! If you had saved up and spent your own money the car would have cost you USD 5 000.
Now you borrowed and paid USD 6 250.
Example:
Let’s say you borrow money and you need EUR 25 000. You borrow this with a simple interest rate of 6%/year but you only need the money for 3 months after which you will pay the amount back fully. What is the total amount accrued and what is the interest you owe?
This may seem as more complicated but once again it is very easy. Just plug in the values in the simple interest formula.
A = P(1 + rt)
A = 25 000(1 + 0.06*(1/4))
Here r is the 6% simple interest in decimal form and t is time BUT in 3 months and not 1 year = 3/12 = ¼
A = 25 000(1 + 0.015)
A = 25 000(1.0150)
A = 25 375
So, your total amount accrued in interest and principal amount borrowed is EUR 25 375 and your simple interest is EUR 25 375 – 25 000 = EUR 375.
IMPORTANT: You could just as well have borrowed money for 1 month in which case time i.e. t would have been 1/12 or 2 days or 26 days in which case time (t) would have been 2/365 or 26/365.
It doesn’t matter what your time period is, with this formula you can calculate the simple interest always.
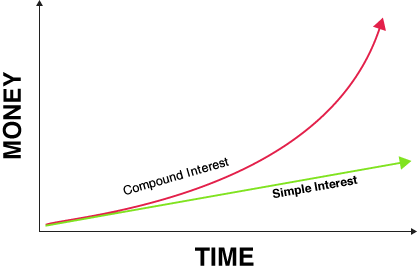
There is also something called compound interest but we will not go into that here. You can read more about that in our compound interest formula blog post though! Compound interest practice test questions can be found here!
Recap
In this article we discussed simple interest, what it is and how it is calculated using the simple interest formula. We looked at various examples and looked at the effect of changing the time period from 1 year to a quarter or month or even daily.
Simple interest is one of the core principles to understand in life in general as you will need this in almost everything. Luckily with the knowledge you have gained here this will now be easy for you!
To practice for simple interest questions you should try these simple interest practice tests.


